find-the-largest-area-of-square-inside-two-rectangles

java

python

python3

mysql

mssql

oraclesql

csharp

javascript

typescript

bash

swift

kotlin

dart

golang

ruby

scala

rust

racket

erlang

elixir

pythondata

postgresql

cangjie

Algorithms

There exist <code>n</code> rectangles in a 2D plane with edges parallel to the x and y axis. You are given two 2D integer arrays&nbsp;<code>bottomLeft</code> and <code>topRight</code>&nbsp;where <code>bottomLeft[i] = [a_i, b_i]</code> and <code>topRight[i] = [c_i, d_i]</code> represent&nbsp;the bottom-left and top-right coordinates of the <code>ith</code> rectangle, respectively.

You need to find the maximum area of a square that can fit inside the intersecting region of at least two rectangles. Return <code>0</code> if such a square does not exist.

&nbsp;
Example 1:
<img alt="" src="https://assets.leetcode.com/uploads/2024/01/05/example12.png" style="width: 443px; height: 364px; padding: 10px; background: rgb(255, 255, 255); border-radius: 0.5rem;" />
Input: bottomLeft = [[1,1],[2,2],[3,1]], topRight = [[3,3],[4,4],[6,6]]

Output: 1

Explanation:

A square with side length 1 can fit inside either the intersecting region of rectangles 0 and 1 or the intersecting region of rectangles 1 and 2. Hence the maximum area is 1. It can be shown that a square with a greater side length can not fit inside any intersecting region of two rectangles.

Example 2:
<img alt="" src="https://assets.leetcode.com/uploads/2024/07/15/diag.png" style="width: 451px; height: 470px; padding: 10px; background: rgb(255, 255, 255); border-radius: 0.5rem;" />
Input: bottomLeft = [[1,1],[1,3],[1,5]], topRight = [[5,5],[5,7],[5,9]]

Output: 4

Explanation:

A square with side length 2 can fit inside either the intersecting region of rectangles 0 and 1 or the intersecting region of rectangles 1 and 2. Hence the maximum area is <code>2 * 2 = 4</code>. It can be shown that a square with a greater side length can not fit inside any intersecting region of two rectangles.

Example 3:
<code> <img alt="" src="https://assets.leetcode.com/uploads/2024/01/04/rectanglesexample2.png" style="padding: 10px; background: rgb(255, 255, 255); border-radius: 0.5rem; width: 445px; height: 365px;" /> </code>

Input: bottomLeft = [[1,1],[2,2],[1,2]], topRight = [[3,3],[4,4],[3,4]]

Output: 1

Explanation:

A square with side length 1 can fit inside the intersecting region of any two rectangles. Also, no larger square can, so the maximum area is 1. Note that the region can be formed by the intersection of more than 2 rectangles.

Example 4:
<code> <img alt="" src="https://assets.leetcode.com/uploads/2024/01/04/rectanglesexample3.png" style="padding: 10px; background: rgb(255, 255, 255); border-radius: 0.5rem; width: 444px; height: 364px;" /> </code>

Input:&nbsp;bottomLeft = [[1,1],[3,3],[3,1]], topRight = [[2,2],[4,4],[4,2]]

Output: 0

Explanation:

No pair of rectangles intersect, hence, the answer is 0.

&nbsp;
Constraints:

<ul>
	<li><code>n == bottomLeft.length == topRight.length</code></li>
	<li><code>2 &lt;= n &lt;= 103</code></li>
	<li><code>bottomLeft[i].length == topRight[i].length == 2</code></li>
	<li><code>1 &lt;= bottomLeft[i][0], bottomLeft[i][1] &lt;= 107</code></li>
	<li><code>1 &lt;= topRight[i][0], topRight[i][1] &lt;= 107</code></li>
	<li><code>bottomLeft[i][0] &lt; topRight[i][0]</code></li>
	<li><code>bottomLeft[i][1] &lt; topRight[i][1]</code></li>
</ul>

largestSquareArea

Find the Largest Area of Square Inside Two Rectangles

Rectangle Area

rectangle-area

矩形面积

Geometry

Array

Math

在二维平面上存在 <code>n</code> 个矩形。给你两个下标从 0 开始的二维整数数组 <code>bottomLeft</code> 和 <code>topRight</code>，两个数组的大小都是 <code>n x 2</code> ，其中 <code>bottomLeft[i]</code> 和 <code>topRight[i]</code> 分别代表第 <code>i</code> 个矩形的 左下角 和 右上角 坐标。

我们定义 向右 的方向为 x 轴正半轴（x 坐标增加），向左 的方向为 x 轴负半轴（x 坐标减少）。同样地，定义 向上 的方向为 y 轴正半轴（y 坐标增加），向下 的方向为 y 轴负半轴（y 坐标减少）。

你可以选择一个区域，该区域由两个矩形的 交集&nbsp;形成。你需要找出能够放入该区域 内 的 最大 正方形面积，并选择最优解。

返回能够放入交集区域的正方形的 最大 可能面积，如果矩形之间不存在任何交集区域，则返回 <code>0</code>。

&nbsp;

示例 1：
<img alt="" src="https://assets.leetcode.com/uploads/2024/01/05/example12.png" style="width: 443px; height: 364px; padding: 10px; background: rgb(255, 255, 255); border-radius: 0.5rem;" />
<pre>
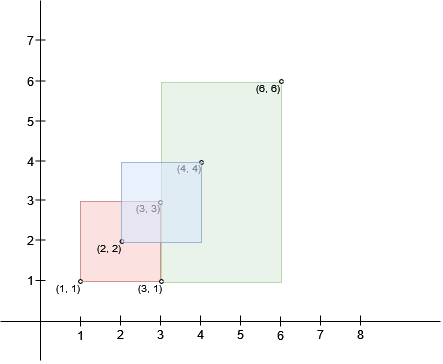
输入：bottomLeft = [[1,1],[2,2],[3,1]], topRight = [[3,3],[4,4],[6,6]]
输出：1
解释：边长为 1 的正方形可以放入矩形 0 和矩形 1 的交集区域，或矩形 1 和矩形 2 的交集区域。因此最大面积是边长 * 边长，即 1 * 1 = 1。
可以证明，边长更大的正方形无法放入任何交集区域。
</pre>

示例 2：
<img alt="" src="https://assets.leetcode.com/uploads/2024/01/04/rectanglesexample2.png" style="padding: 10px; background: rgb(255, 255, 255); border-radius: 0.5rem; width: 445px; height: 365px;" />
<pre>
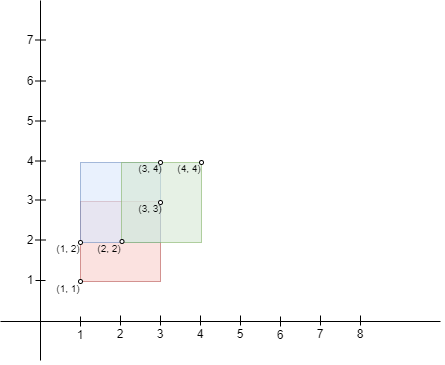
输入：bottomLeft = [[1,1],[2,2],[1,2]], topRight = [[3,3],[4,4],[3,4]]
输出：1
解释：边长为 1 的正方形可以放入矩形 0 和矩形 1，矩形 1 和矩形 2，或所有三个矩形的交集区域。因此最大面积是边长 * 边长，即 1 * 1 = 1。
可以证明，边长更大的正方形无法放入任何交集区域。
请注意，区域可以由多于两个矩形的交集构成。
</pre>

示例 3：
<img alt="" src="https://assets.leetcode.com/uploads/2024/01/04/rectanglesexample3.png" style="padding: 10px; background: rgb(255, 255, 255); border-radius: 0.5rem; width: 444px; height: 364px;" />
<pre>
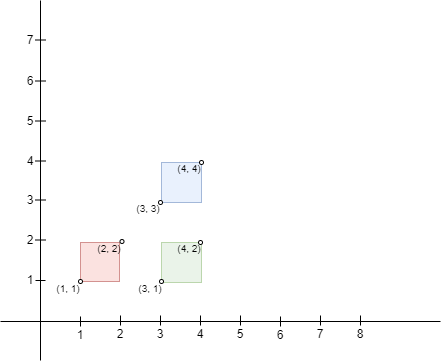
输入：bottomLeft = [[1,1],[3,3],[3,1]], topRight = [[2,2],[4,4],[4,2]]
输出：0
解释：不存在相交的矩形，因此，返回 0 。
</pre>

&nbsp;

提示：

<ul>
	<li><code>n == bottomLeft.length == topRight.length</code></li>
	<li><code>2 &lt;= n &lt;= 103</code></li>
	<li><code>bottomLeft[i].length == topRight[i].length == 2</code></li>
	<li><code>1 &lt;= bottomLeft[i][0], bottomLeft[i][1] &lt;= 107</code></li>
	<li><code>1 &lt;= topRight[i][0], topRight[i][1] &lt;= 107</code></li>
	<li><code>bottomLeft[i][0] &lt; topRight[i][0]</code></li>
	<li><code>bottomLeft[i][1] &lt; topRight[i][1]</code></li>
</ul>